A) ![]()
B) ![]()
C) ![]()
D) ![]()
E) ![]()
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Set up and evaluate a double integral to find the volume of the solid bounded by the graphs of the equations given below. 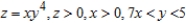
A) ![]()
B) ![]()
C) ![]()
D) ![]()
E) ![]()
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Use a double integral in polar coordinates to find the volume of the solid inside the hemisphere 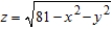 but outside the cylinder
but outside the cylinder 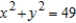 .
.
A) ![]()
B) ![]()
C) ![]()
D) ![]()
E) ![]()
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Evaluate the following iterated integral. 
A) ![]()
B) ![]()
C) ![]()
D) ![]()
E) ![]()
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Use a change of variables to find the volume of the solid region lying below the surface 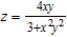 and above the plane region R: region bounded by the graphs of
and above the plane region R: region bounded by the graphs of  (Hint: Let
(Hint: Let 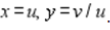 .) Round your answer to two decimal places.
.) Round your answer to two decimal places.
A) 5.13
B) 21.35
C) 3.56
D) 5.13.
E) 42.70
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Find the mass of the lamina described by the inequalities 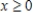 and
and 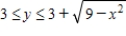 , given that its density is
, given that its density is 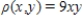 .
(Hint: Some of the integrals are simpler in polar coordinates.)
.
(Hint: Some of the integrals are simpler in polar coordinates.)
A) ![]()
B) ![]()
C) ![]()
D) ![]()
E) ![]()
Correct Answer

verified
A
Correct Answer
verified
Multiple Choice
Evaluate the double integral below. 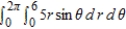
A) ![]()
B) 0
C) ![]()
D) ![]()
E) ![]()
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Set up a triple integral that gives the moment of inertia about the 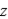 -axis of the solid region Q of density given below.
-axis of the solid region Q of density given below. 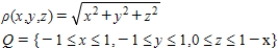
A) ![]()
B) ![]()
C) ![]()
D) ![]()
E) ![]()
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Set up an integral for both orders of integration, and use the more convenient order to evaluate the integral below over the region R. 
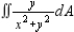

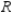
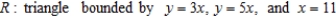
A) ![]()
B) ![]()
C) ![]()
D) ![]()
E) ![]()
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Evaluate the iterated integral 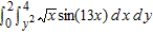 by switching the order of integration. Round your to three decimal places.
by switching the order of integration. Round your to three decimal places.
A) 0.056
B) 0.076
C) 36.056
D) 4.056
E) 3.056
Correct Answer

verified
A
Correct Answer
verified
Multiple Choice
Use a change of variables to find the volume of the solid region lying below the surface 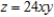 and above the plane region R: region bounded by the square with vertices
and above the plane region R: region bounded by the square with vertices 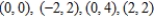 .
.
A) ![]()
B) ![]()
C) ![]()
D) ![]()
E) ![]()
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Find the mass of the triangular lamina with vertices 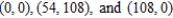 for the density
for the density 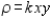 .
.
A) 11,337,408k
B) 22,674,826k
C) 11,337,398k
D) 11,337,413k
E) 22,674,816k
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Set up and evaluate a double integral to find the volume of the solid bounded by the graphs of the equations 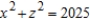 and
and 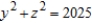 in the first octant.
in the first octant.
A) 273,375
B) 30,375
C) 182,250
D) 91,125
E) 60,750
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Use cylindrical coordinates to find the volume of the solid inside both 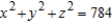 and
and 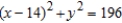 .
.
A) ![]()
B) ![]()
C) ![]()
D) ![]()
E) ![]()
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Use an iterated integral to find the area of the region shown in the figure below. 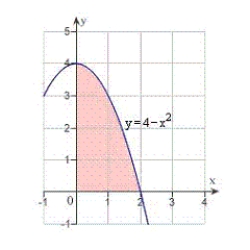
A) ![]()
B) ![]()
C) ![]()
D) ![]()
E) ![]()
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Write a double integral that represents the surface area of 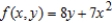 over the region R: triangle with vertices
over the region R: triangle with vertices 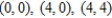 . Use a computer algebra system to evaluate the double integral. Round your answer to two decimal places.
. Use a computer algebra system to evaluate the double integral. Round your answer to two decimal places.
A) ![]()
B) ![]()
C) ![]()
D) ![]()
E) ![]()
Correct Answer

verified
B
Correct Answer
verified
Multiple Choice
Find the center of mass of the lamina bounded by the graphs of the equations 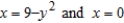 for the density
for the density 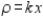 .
.
A) ![]()
B) ![]()
C) ![]()
D) ![]()
E) ![]()
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Use a triple integral to find the volume of the solid bounded by the graphs of the equations 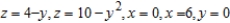 .
.
A) ![]()
B) ![]()
C) ![]()
D) ![]()
E) ![]()
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Evaluate the iterated integral 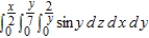 .
.
A) ![]()
B) ![]()
C) ![]()
D) ![]()
E) ![]()
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Use a double integral to find the volume of the indicated solid. 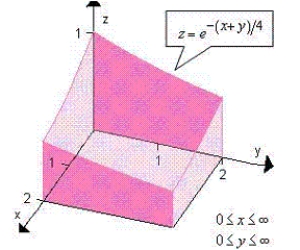
A) 16
B) 9
C) 4
D) 20
E) 6
Correct Answer

verified
Correct Answer
verified
Showing 1 - 20 of 143
Related Exams